Find The Value Of 8

Example 27 - Chapter 3 Class xi Trigonometric Functions (Term two)

Last updated at February. xiii, 2020 by Teachoo

This video is only bachelor for Teachoo blackness users

---

Transcript

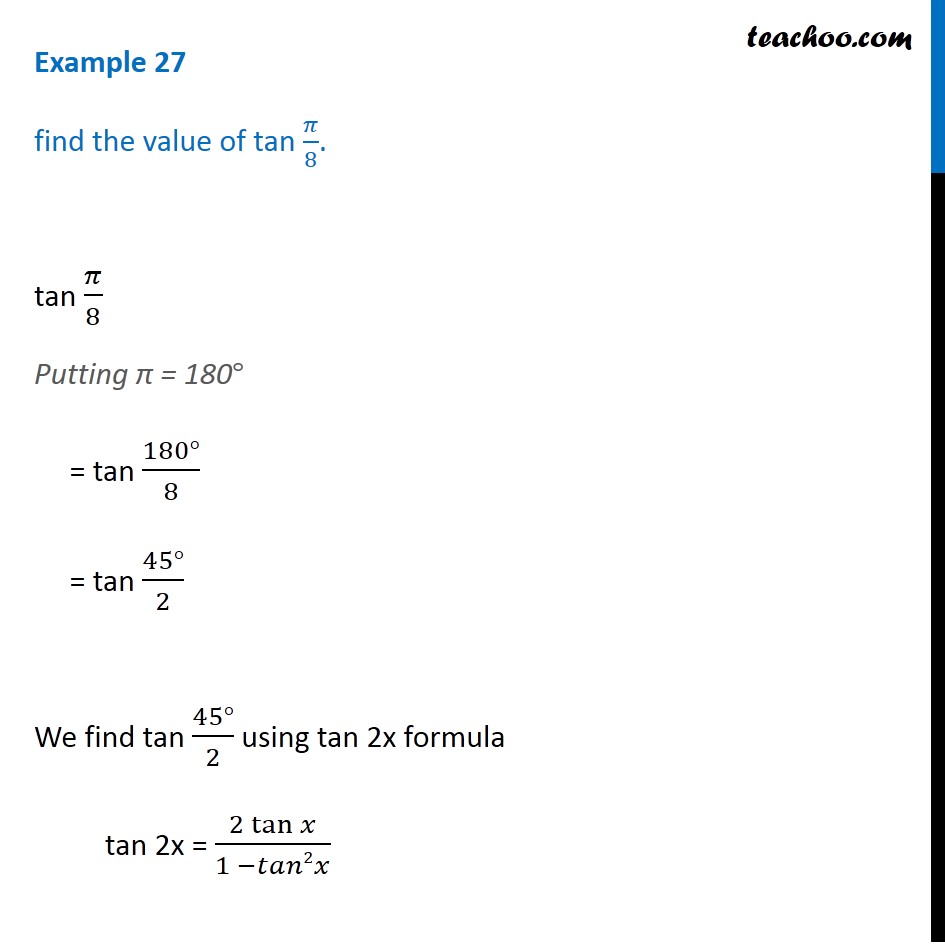
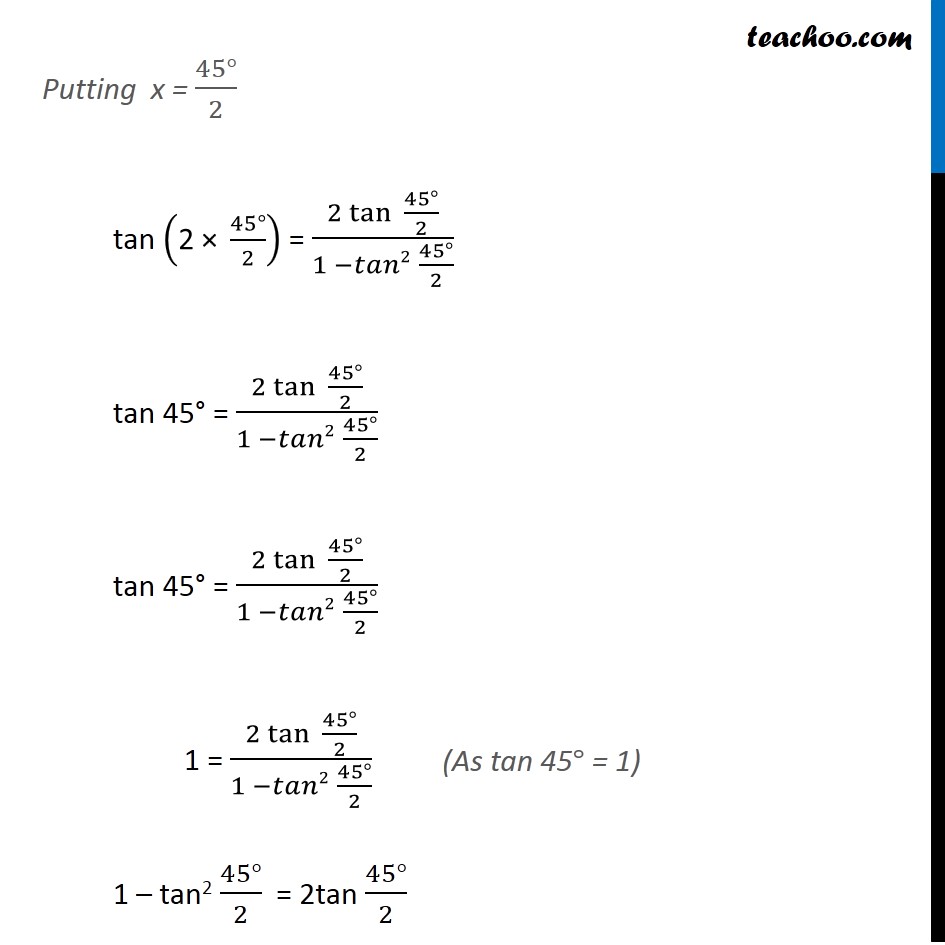
Instance 27 find the value of tan 𝜋/8. tan 𝜋/8 Putting π = 180° = tan (180°)/8 = tan (45°)/ii We detect tan (45°)/2 using tan 2x formula tan 2x = (2 tan⁡𝑥)/(1 −𝑡𝑎𝑛2𝑥) Putting ten = (45°)/2 tan ("2 × " (45°)/two) = (two tan⁡〖 (45°)/two〗)/(1 −𝑡𝑎𝑛2 (45°)/ii) tan 45° = (two tan⁡〖 (45°)/2〗)/(1 −𝑡𝑎𝑛two (45°)/2) tan 45° = (ii tan⁡〖 (45°)/ii〗)/(i −𝑡𝑎𝑛2 (45°)/2) 1 = (2 tan⁡〖 (45°)/2〗)/(1 −𝑡𝑎𝑛ii (45°)/two) 1 – tan2 (45°)/2 = 2tan (45°)/two (As tan 45° = 1) Let tan (𝟒𝟓°)/𝟐 = x So, our equation becomes 1 – x2 = 2x 0 = 2x + x2 – ane x2 + 2x – 1 = 0 The higher up equation is of the form ax2 + bx + c = 0 where a = one, b = 2, c = −i Solution are x = (− 𝑏 ± √(𝑏ii −4𝑎𝑐) )/two𝑎 = (− 2 ± √((−ii)two − 4 × one × (−1)) )/(2 × 1) = (−two ± √(4 + 4))/2 = (−ii ± √8)/2 = (−2 ± √(2 × ii × ii))/2 = (−two ± two√2)/2 = (two ( −1 ±√2 ))/2 = –i ± √2 Thus, ten = –one ± √two tan (45°)/ii = –1 ± √two But tan (45°)/2 = –i – √two is not possible as (45°)/2 = 22.five° lies in first quadrant & tan is positive in showtime quadrant Therefore, tan (45°)/ii = −1 + √2 i.east. tan 𝝅/𝟖 = √𝟐 – ane

Find The Value Of 8,

Source: https://www.teachoo.com/2231/586/Example-27---Find-value-of-tan-pi-8---Chapter-3-Class-11/category/Examples/

Posted by: heywardforthishe.blogspot.com

Share this post